Chaos (from Greek cháos "primal emptiness"): a state of systems characterized by extreme unpredictability or susceptibility to influence.
In Greek mythology, chaos was the primal state of the world from which the ►gods were generated. In later times, "chaos" acquired a connotation of complete disorder in contrast to ►cosmos, the orderly world. Contrary to popular views, there is no scientific "chaos theory". However, subdisciplines of mathematics and physics that deal with the development of so-called non-linear systems are sometimes called "chaos theory".
"Chaos" refers less to the order or disorder of a system and more to its behavior. Any small changes in the initial state of a non-linear system may lead to drastic and changes in its later development that are unpredictable in practice. Such a system is also characterized by an almost unlimited sensitivity to external factors. This is illustrated by the famous butterfly effect: "A butterfly flapping its wings in the Amazon jungle can cause a hurricane in Europe."
Indeed, the weather is a classic example of a non-linear system. In 1963, the meteorologist Edward Lorenz developed a simple computer model to predict pressure and temperature in the atmosphere. In doing so, he discovered that tiny changes in the intial parameters of his equations lead to vastly different weather effects. To describe this phenomenon, he introduced the term "butterfly effect". The system that he chose for his model consists of three relatively simple ►functions - differential equations* - and generates a chaotic mathematical curve called the Lorenz attractor.
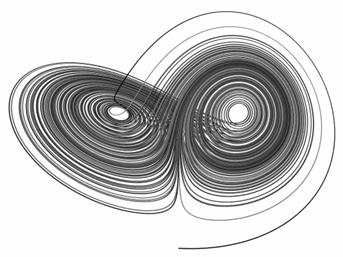
Lorenz attractor
The butterfly effect is neither due to chance nor related in any way to the ►uncertainty principle in quantum physics. Though it can be triggered by genuinely chance events such as quantum fluctuations, it also occurs in completely deterministic systems whose behavior is not affected by coincidences. Simple physical systems, such as turbulence in a liquid or a pendulum connected to another pendulum, can display chaotic behavior with extreme sensitivity to minute alterations of their initial state. Certain mathematical curves, especially the ►fractales, display this effect as well.
Though coincidence does not really play a part in such systems, to us it appears to do so because the effect of unnoticeable changes in initial conditions cannot be predicted. The butterfly effect also occurs in much more complex non-linear systems such as history: After all, the wrong turn taken by a driver in Sarajevo triggered World War I.
* dx/dt = a(y-x)
dy/dt
= x(b-z) - y
dz/dt
= xy - cz
The numerical solution of these three equations reveals chaotic behavior in certain parameter values such as a = 10, b = 28, c = 2.666.