Fractal (from Latin fractus, "broken"): an object of infinite roughness.
The structure of a fractal object is reiterated in its magnifications. Ideal fractals can be magnified indefinitely without losing their structure and becoming "smooth". In this sense, they have an infinitely rough structure or surface.
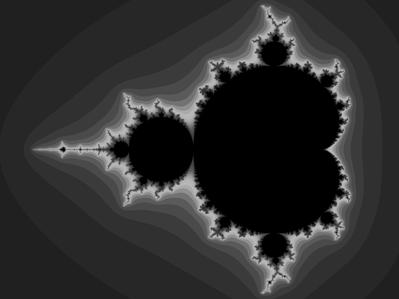
The term "fractal" was first coined in 1975 by the mathematician Benoit Mandelbrot. The fractal that he famously introduced is the so-called Mandelbrot set (see illustration), that is, the set of all numbers c for which the Mandelbrot function M(n,c) does not increase beyond all limits with increasing value of n. The Mandelbrot function is a recursive ►function defined as follows:
M(0,c) = 0
M(n,c) = M(n-1,c)2 + c
c is a complex number consisting of two numerical components, namely, a real number and a multiple of the square root of -1. In the above image, the grey-colored areas in the regions of (-1.5 ... 0.5) and (-1 ... 1) of the two numerical components of c represent the value of the Mandelbrot function. The bulb-shaped structures along the margin contain scaled-down copies of the Mandelbrot set. Each bulb in turn generates smaller bulbs. Thus, each margin section contains infinitely many of these bulbs at any given scale. This characteristic of fractal sets is called "self-similarity". As you can see, this quite simple function can be used to generate complicated and beautiful images.
There are many mathematical functions that generate fractal curves by recursively replacing parts of a figure with the figure itself. There are also fractals outside the realm of mathematics. If you look at a head of Romanesco broccoli (see image) before eating it, you will notice typical fractal structures. The Romanesco broccoli displays spiral-shaped bulbs that consist in turn of more spiral-shaped bulbs and are arranged in spirals on the broccoli's surface.

Fractal Vegetable
Fractals can be used to assess audacious claims such as "Norway's coastline is of infinite length". As we know, Norway has an extremely rugged coastline with many fjords, which makes it an ideal candidate for a fractal. Indeed, the fjords in turn contain secondary fjords that branch off into tertiary fjords, and so forth. The more closely we inspect the coastline, the more its observed length will increase — up to the microscopic cracks in the cliff rocks on shore. If the size of the rock's particles, or that of atoms in general, were not finite then Norway would indeed possess an infinite coastline.